Author : Matthias Weber in colaboration with, Malcolm Mobutu Smith.
Word from the editor: Matter Meddles with Math was part of a project at Indiana University (Bloomington) with the name “(Re)Imagining Science”, where artists and scientists would collaborate on artistic rendering of scientific objects. The outcome was a small exhibit with works from eight teams, it will be shown early 2018 in Philadelphia.
Matter Meddles with Math
While artists create objects by making them, mathematicians create objects by proving that they exist. As a consequence, mathematicians and artists often value quite different aspects of the same object. For instance, an ensō might appeal to the mathematician as the representation of a perfect circle, while the artist is fascinated by the irregularities of the brush stroke.
We have engaged in a dialogue about these values, trying to transfer abstract mathematical ideas about shape into a compelling reality. We have brought together expertise in bending and folding plastics (Martha MacLeish), clay sculpting (Malcolm Smith), and minimal surface geometry (Matthias Weber).
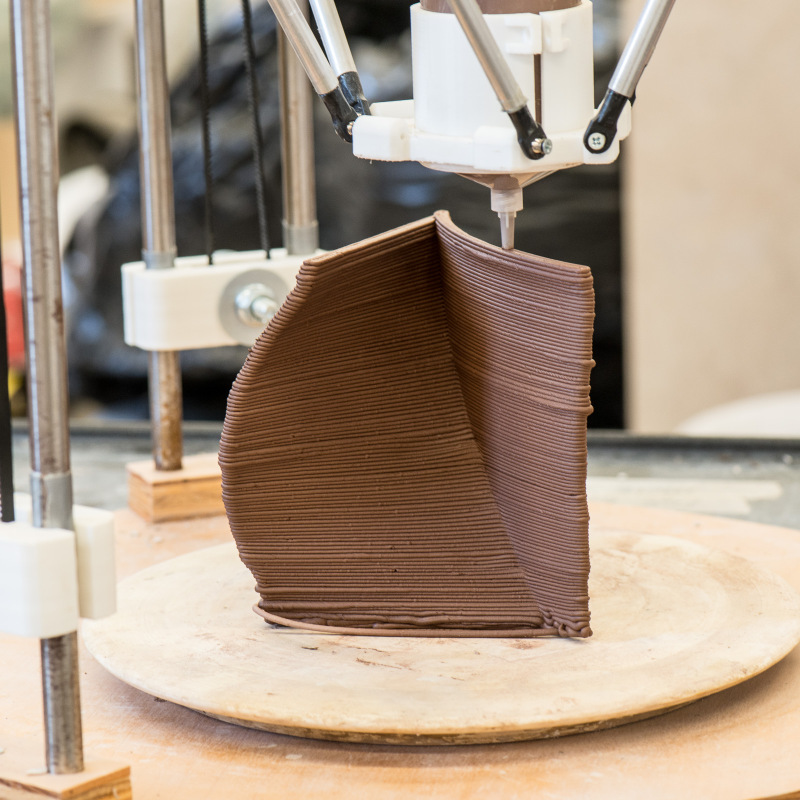
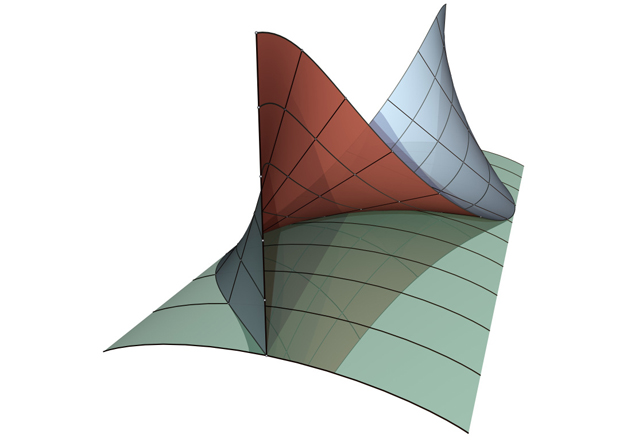
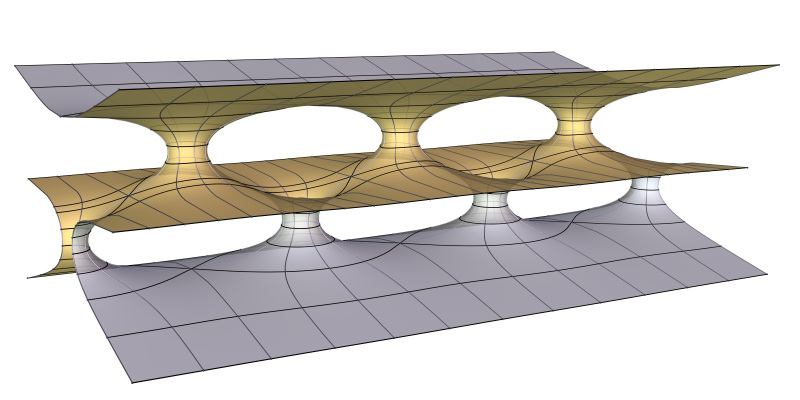
It quickly became clear that we would not just take a mathematical object and make it. The materials and building techniques we have available impose severe constraints on what is possible to build. For instance, objects that are bent out of stiff material are mathematically flat, while the minimal surfaces we wanted to present certainly aren’t. This lead to intense discussions about among the how various mathematical ways to represent the same object, only some were realizable by our means. An example is our realization of the gyroid minimal surface: Instead of displaying the curved surface, we devised a modular construction of its skeletal graph whose elements are curved shapes that we could construct. Or, instead of representing a curved minimal surface, we show its net of curvature lines through ruled surfaces perpendicular to the minimal surfaces.


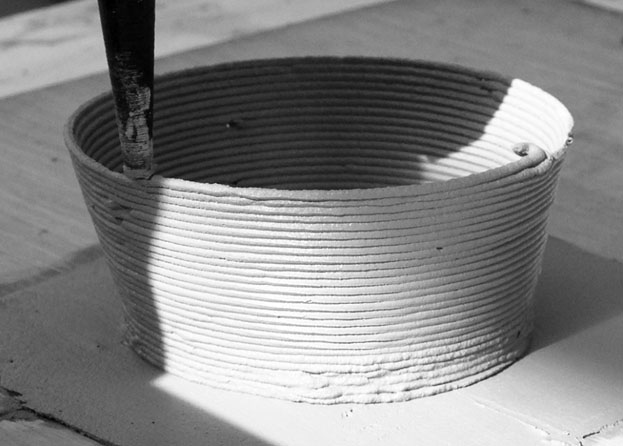
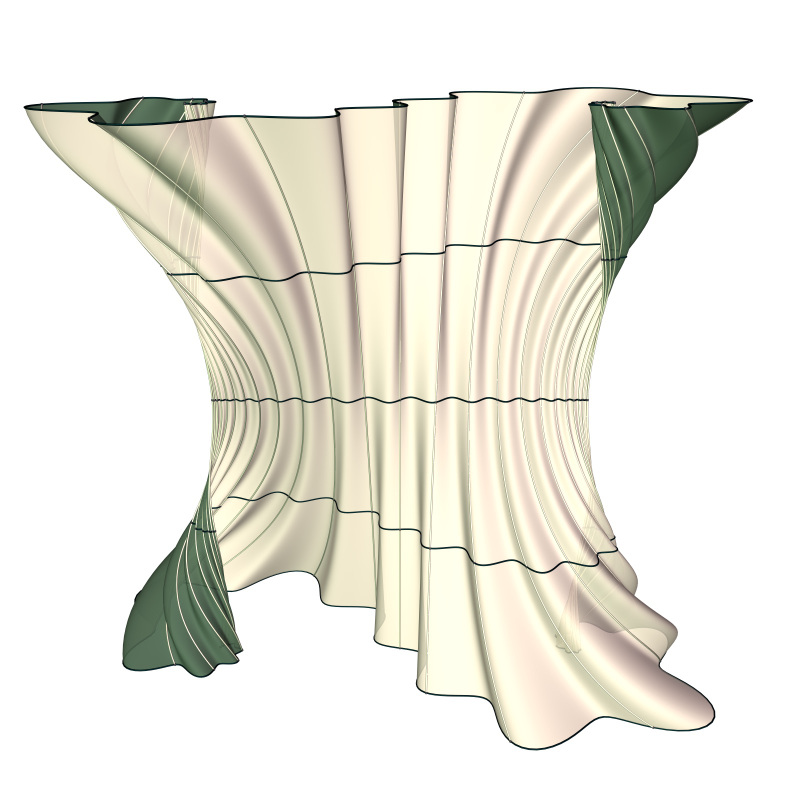
Similarly, Malcolm decided to build a clay printer. The illusion this machine could be used to create ceramics objects of any imaginable type soon dissolved. Our clay printer creates objects out of a single, continuous thread of clay, layer by layer. This lead us to explore how 1-dimensional shapes can be changed layer by layer in order to create meaningful 2-dimensional surfaces. We did this using the simplest geometric shapes: Lines and circles. Navigating the intricacies of the clay printer has become a theme for us, and our first sculptures can be tied to the theme of navigation in general. In our final two clay sculptures we display modern variations of a classical minimal surface; here we have pushed the process of clay printing to its limit.
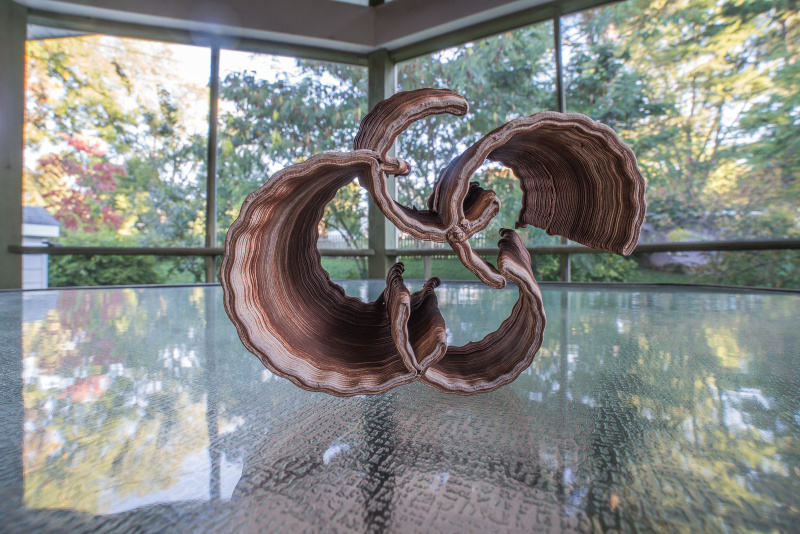

In all our objects we had to take away from the abstract purity of a mathematical shape and to embrace imperfections of material and process. Instead of cosmetically covering up the blemishes, we decided to emphasize them to the extent that we went back to the mathematical model and even changed their theoretical underpinnings. For instance, in the Drunken Boat, we superposed the perfect mathematical loxodrome that we could not print in its ideal shape with a dampened sine function.

The result are objects that show that in reality we can achieve our aims only by embracing imperfectness.
“Instead of getting exactly what I had in mind, the question became what shapes are actually possible to print. At this time, the project is finished, but I certainly want to return to it, likely programming directly in gcode (or rather, exporting gcode from Mathematica).” Matthias Told us.
Source and Pictures : The inner Frame